Using Mental Math strategies means solving a math problem mentally using a conceptual understanding of the numbers. Teaching Mental math Strategies to students is very important as it develops their flexible thinking and boosts their confidence in math. Being able to use the multiplication properties to apply mental math strategies shows a deep conceptual understanding that will, later on, be applied to more advanced concepts.
Some students discover mental math strategies through practice, naturally, however, most of the students need to be taught the strategies and practice applying them to selected problems. There is a special joy in discovering a strategy, a “trick”. We can guide our students to “discover” a strategy by providing appropriate problems and discussing them.
Encouraging students to look for patterns and connections in multiplication and finding quicker methods to solve problems will help them develop their mental multiplication skills and improve their number sense. Apart from the algorithm that requires splitting a number according to place value, there are many more strategies that students can apply to solve problems in different ways. Find the mental math strategies- Addition and subtraction here.
Properties
Mental Math strategies come from using the properties of the numbers and operations in our favor, in a way that makes solving the problem “quicker”. Make sure that your students understand the properties of multiplication below.
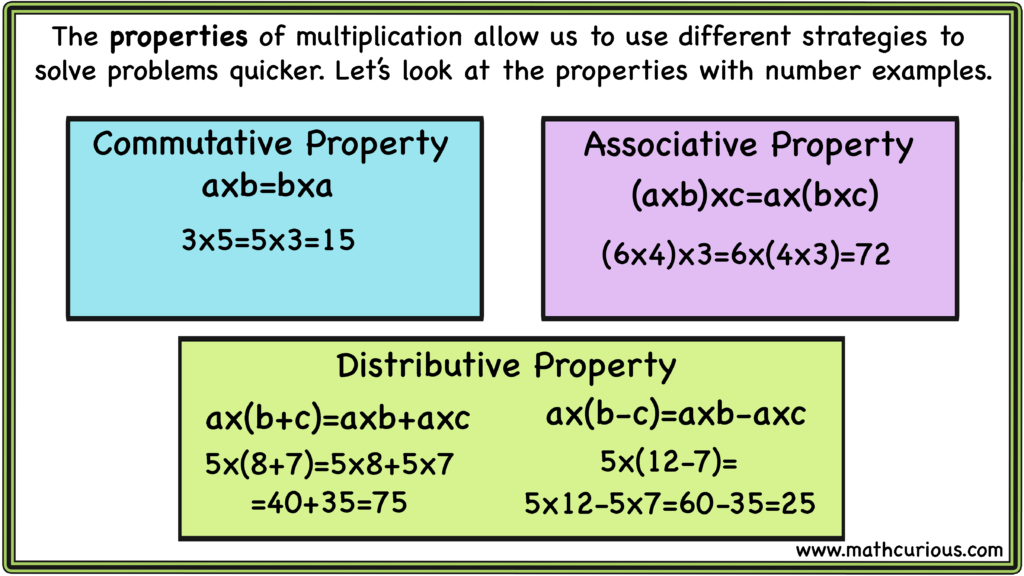
Below are some resources that help students practice properties when they start with multiplication and arrays.
Multiplication and its properties (Building Arrays with Bricks)
Multiplication Mission – arrays, properties, multiples, factors, division
Visuals and Manipulatives
Using visuals and manipulatives reinforces the strategies and helps all students gain a deep understanding. Use base 10 blocks, beads on strings, and other manipulatives to make sure that your students understand the strategies. Use examples with small numbers before you move to greater numbers.
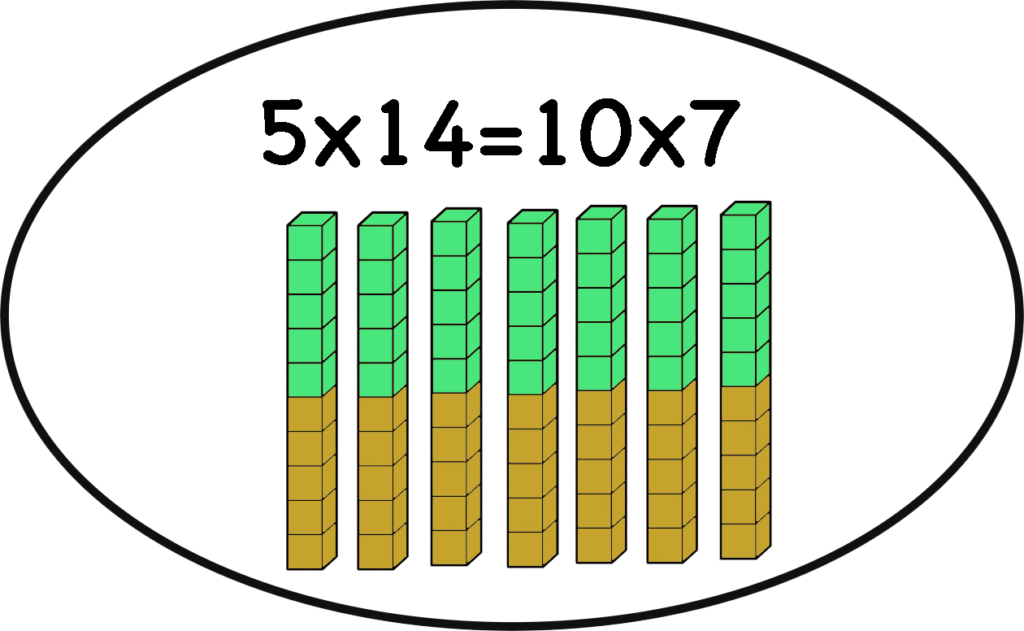
Mental Math strategies as Math Talks
Mental Math strategies make great math talks. Present a problem and ask students how to solve it. Can we do it mentally? Present a solution and ask them to explain. Discuss the different solutions and find the connections. Introduce strategies through math talks and apply them to more problems. Ask questions like: Which mental math strategy is for this problem? Why? I always include mental math strategies in math talks and I find that it helps the students see beyond the algorithm and look for relations in numbers.
Below are some mental math strategies that will make solving problems more fun and more interesting and will expand students’ skills and improve their mental multiplication skills.
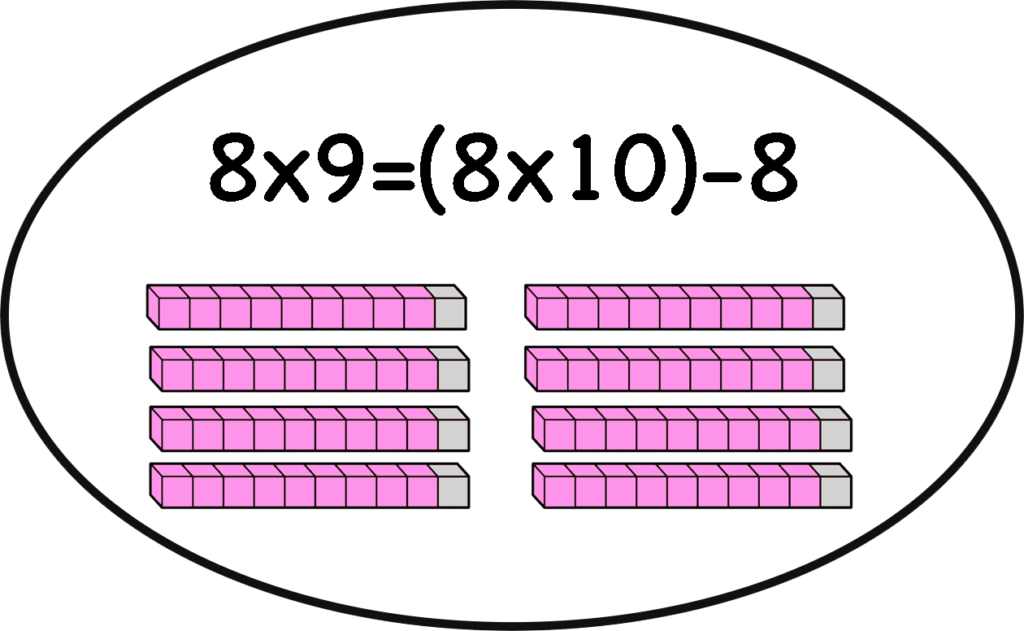
Multiplying by 9 and 11
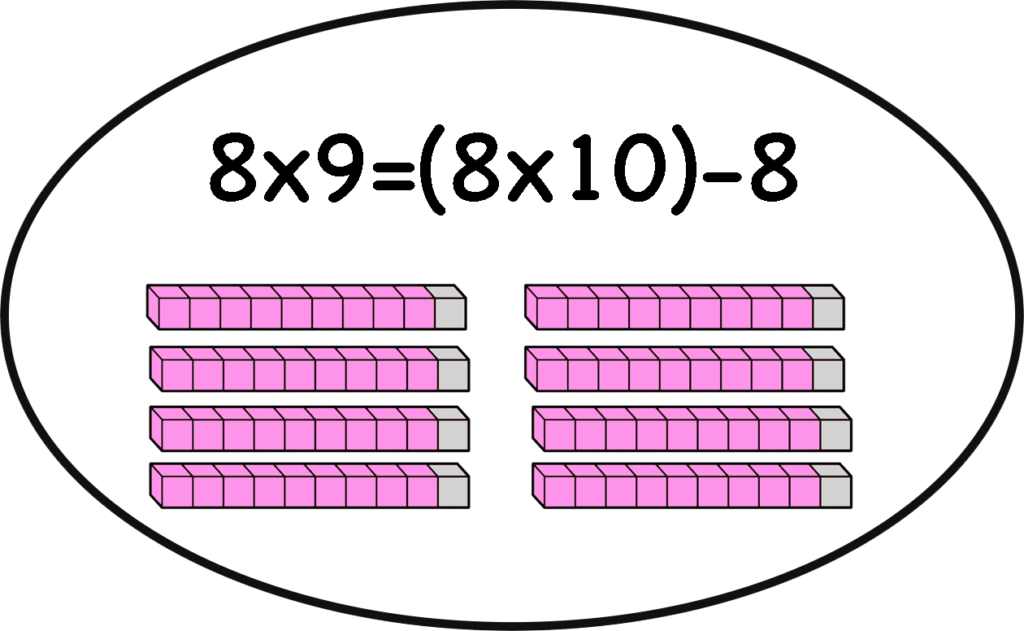
The visual above shows this strategy that makes one of the most tricky multiplication tables easy to figure out quick. When multiplying by 9s we can multiply by 10s and subtract one for each nine multiplied. same way, when multiplying by 11 we can multiply by 10 and add one for each 11 multiplied. This strategy comes in handy when multiplying greater numbers as well. For example, 37×9=37×10-37=370-37=333 Same strategy can be applied when multiplying by 101 or 110.
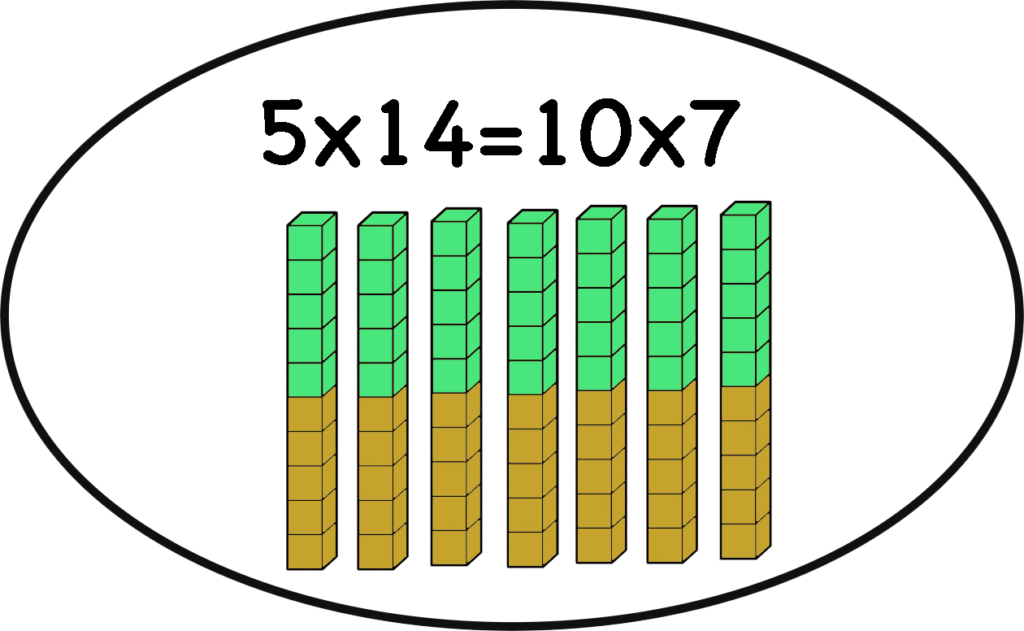
Multiplying by 5 and 4
Multiplying a number by 5 is the same as multiplying its half by 10. 5×54=10×27=270. When multiplying by 4 we can double the number twice and when multiplying by 8 we can double the number thrice.
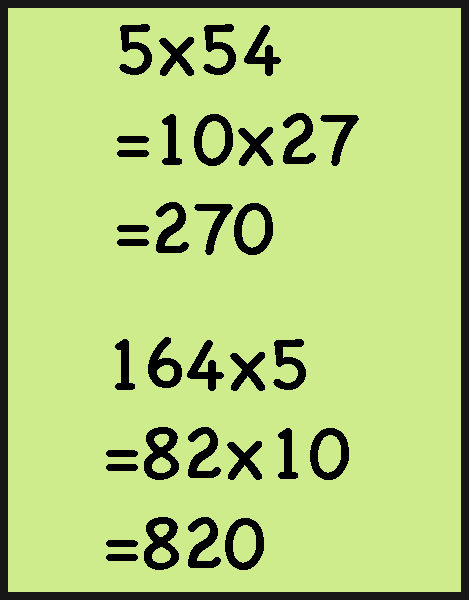
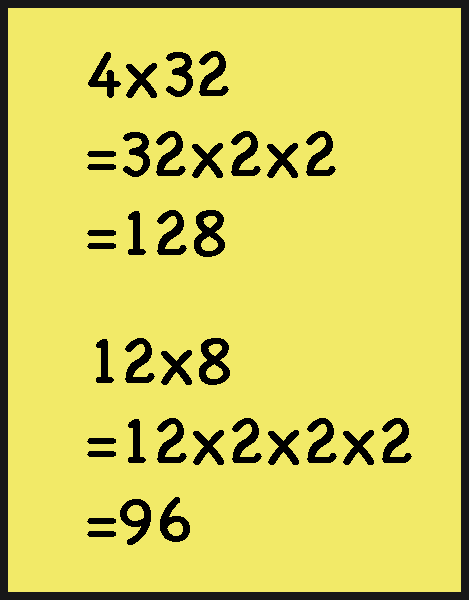
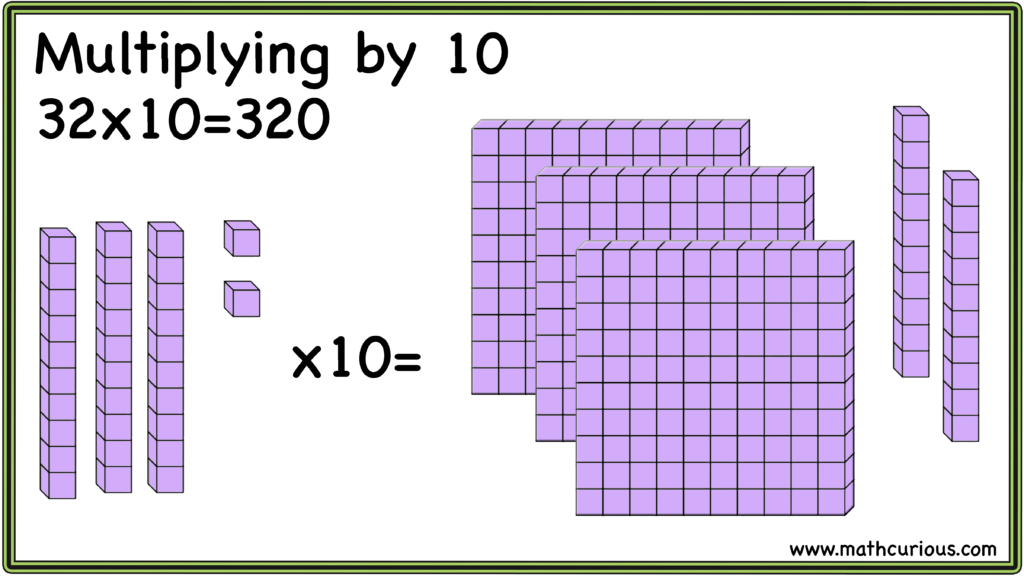
Multiplying by 10,100,1000…
Students need to conceptually understand why this strategy works. Working with base 10 blocks will help students see how when a number is multiplied by 10 the ones become 10s the tens become 100s and so on. Combined with other strategies works well for solving complicated problems.
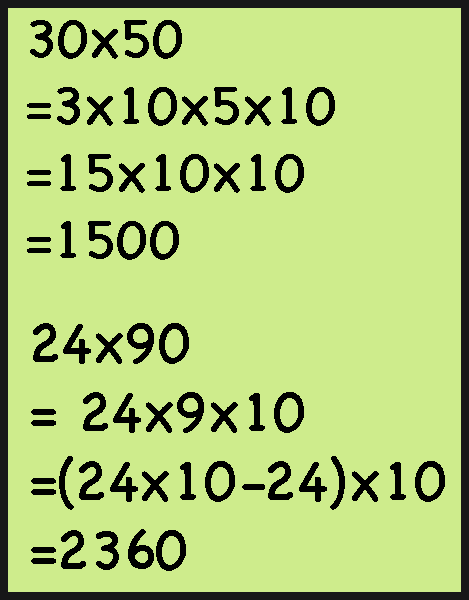
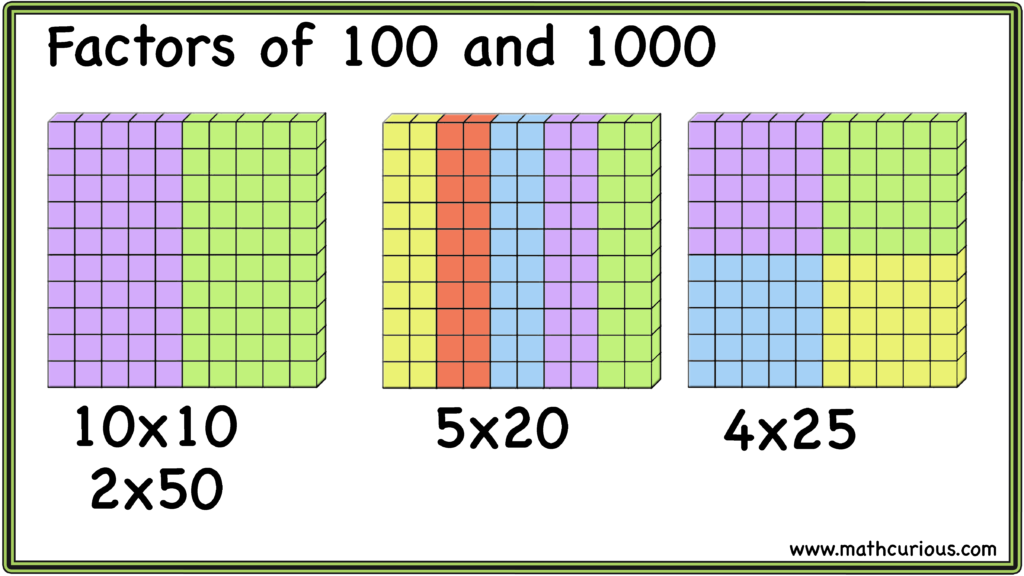
Factors of 100 and 1000-special numbers
Knowing the factors of 100 and 1000 will help students apply strategies such as multiplying in stages and clustering (more about these strategies below) 2×50=100, 4×25=100, 5×20=100, 8×125=1000, and so on. For example,e to multiply by 50 multiply by 100, and divide by 2. To multiply by 25 multiply by 100 and divide by 4.
Grouping/Pairing-Using the associative property
We can pair together numbers that are easier to multiply, or that give us multiples of 10 as products.
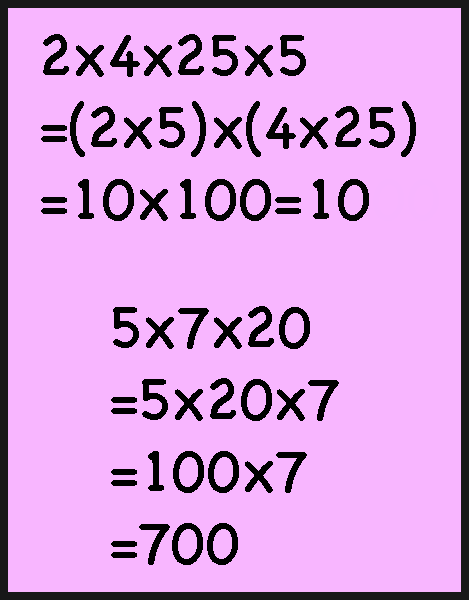
Splitting or multiplying in parts and front-end multiplication.
This strategy is the foundation of the standard multiplication algorithm and it is taught usually in grade 3. A variation of this method is the front-end multiplication where we multiply the biggest quantity first. For example, we multiply the 100s before the tens and the 10s before the ones. Then we add the partial products to get the final product. This strategy works well for estimating and it is based on the distributive property of multiplication over addition.

Multiplying in stages-Factors
We can replace a number with its factors (product of smaller factors) and multiply in stages. Multiplying by 4 can be seen as multiplying by 2 twice, multiplying by 12 is the same as multiplying by 3 and then 4.
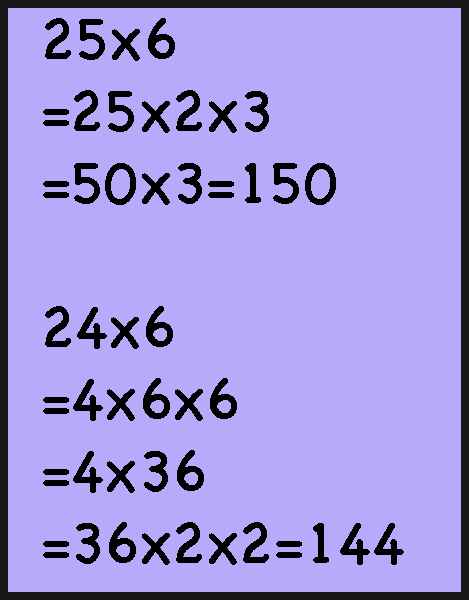
Multiplication by Rounding
We can use this method when one of the numbers is close to a multiple of 10 or for numbers close to a known fact. We round the number to the nearest ten or the known fact and then adjust with addition or subtraction. This strategy uses the distributive property.

Multiplication by halving and doubling
For this strategy, we double one factor and halve the other which leaves the same. We can expand it to solve problems where one of the factors cannot be halved more as well. This strategy is also called the Russian multiplication method and it is very interesting. More about the Russian multiplication method and activities here
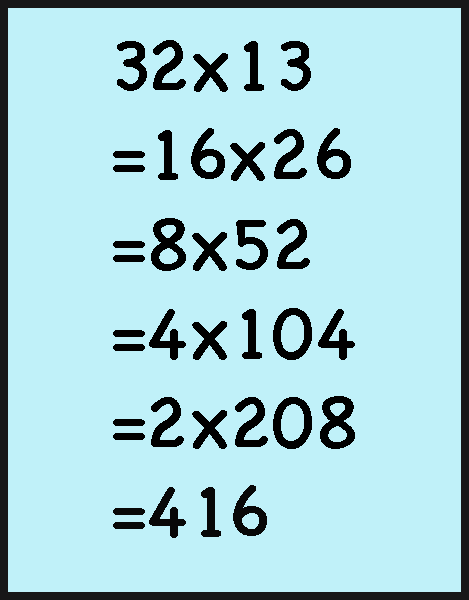
I have created a collection of activity cards to practice the mental math strategies above with visuals, examples, and practice questions. Sample below.
Multiplication Mental Math strategies google slides
Multiplication Mental Math strategies print task cards
Multiplication Mental math strategies worksheets
Bundle of both google slides and print task cards
Bundle of all mental Math strategies, Addition, Subtraction, Multiplication, and Division google slides
Bundle of all mental math strategies subtraction addition, multiplication division worksheets
More strategies resources
Find the mental math strategies- Addition and subtraction here.
Find the Mental Math Strategies -Division here
More ideas
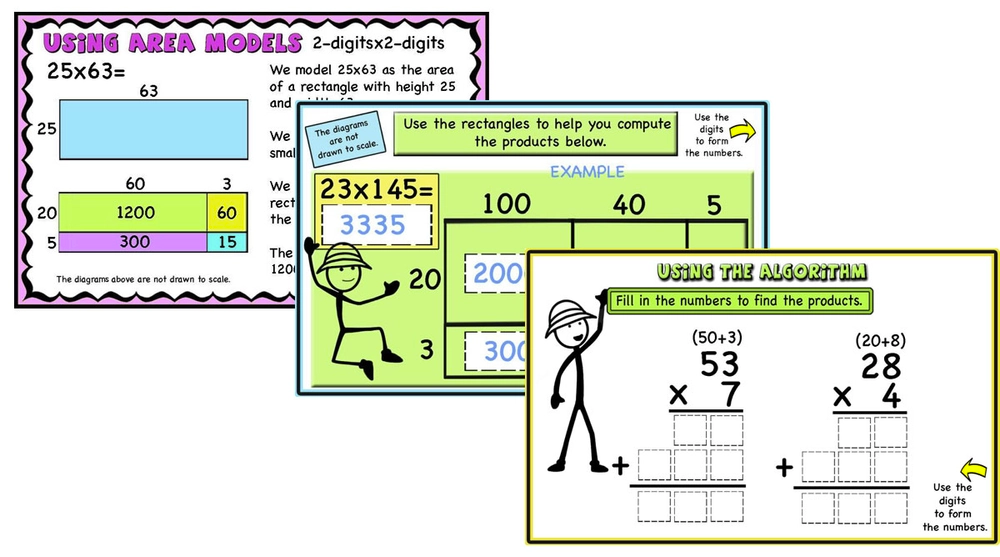
To be able to apply the strategies above to multiplying 2 (and more)-digit numbers, students need to be familiar with the multiplication properties and fluent with the multiplication facts. Here are some simpler strategies and activities to help practice the multiplication facts in a stress-free way and gain fluency.

If you are teaching the multi-digit multiplication algorithm starting with area models here are some ideas and activities.
Multi-Digit Multiplication, Area model, Partial Products algorithm, Puzzles, Word problems