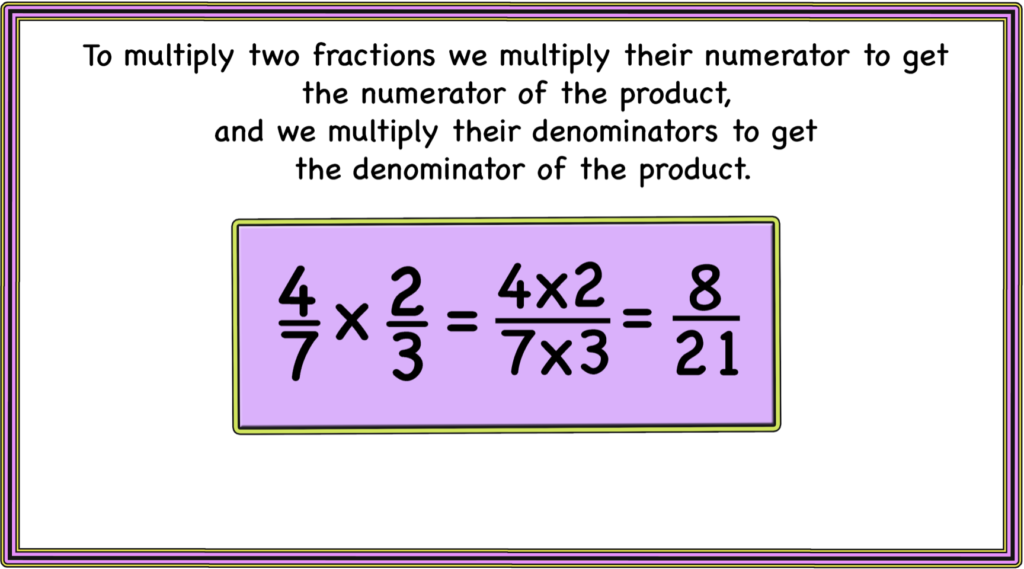
Fractions operations are usually a challenging concept for students to grasp. The multiplication of fractions seems to be “easier” than adding and subtracting fractions since the algorithm is more straightforward in the sense that we do not need to find the LCM and turn the fractions into like ones.
However, it is important the students gain a deep understanding of what it means to multiply fractions. Visual representations like number lines and shape models will help the students better grasp the concept.
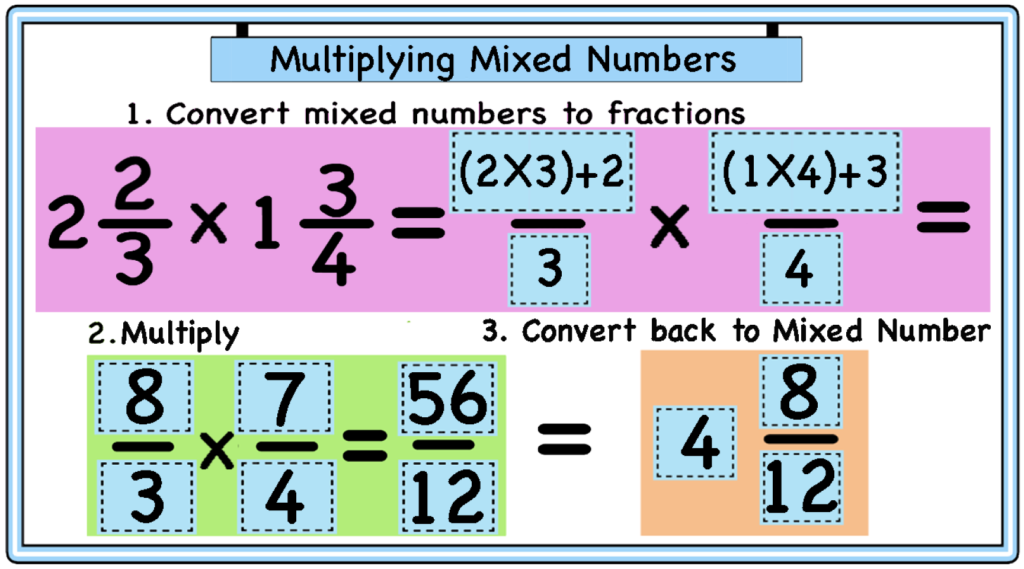
Moreover, other concepts like converting mixed numbers to improper fractions and the opposite, simplifying, and equivalent fractions are connected to multiplying fractions. The most efficient way to multiply mixed numbers is to convert them into improper fractions. Simplifying fractions requires students to understand equivalent fractions and understand the role of prime numbers and GCF.
Below are some ideas and activities to introduce and practice the multiplication of fractions.
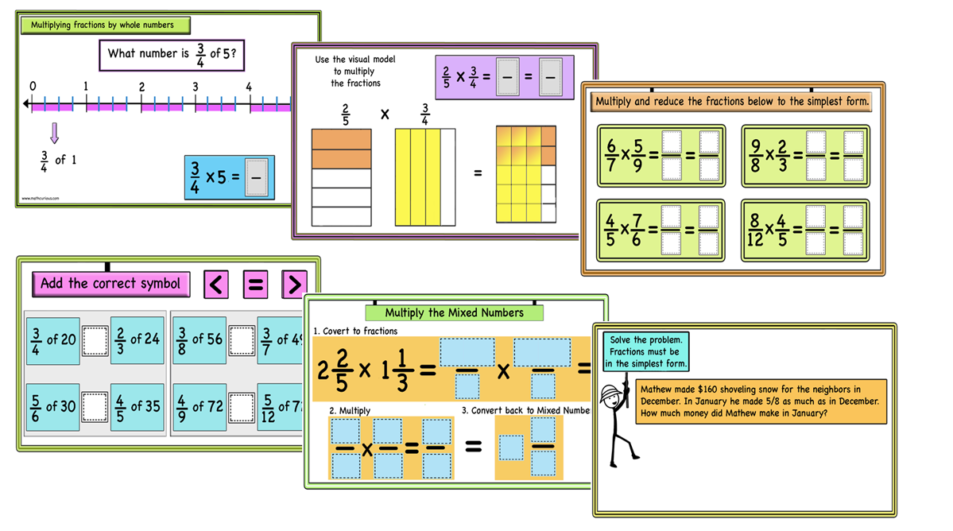
Use number lines, shapes/ area models, and arrays to show that multiplying fractions is finding a part of another part of the whole.
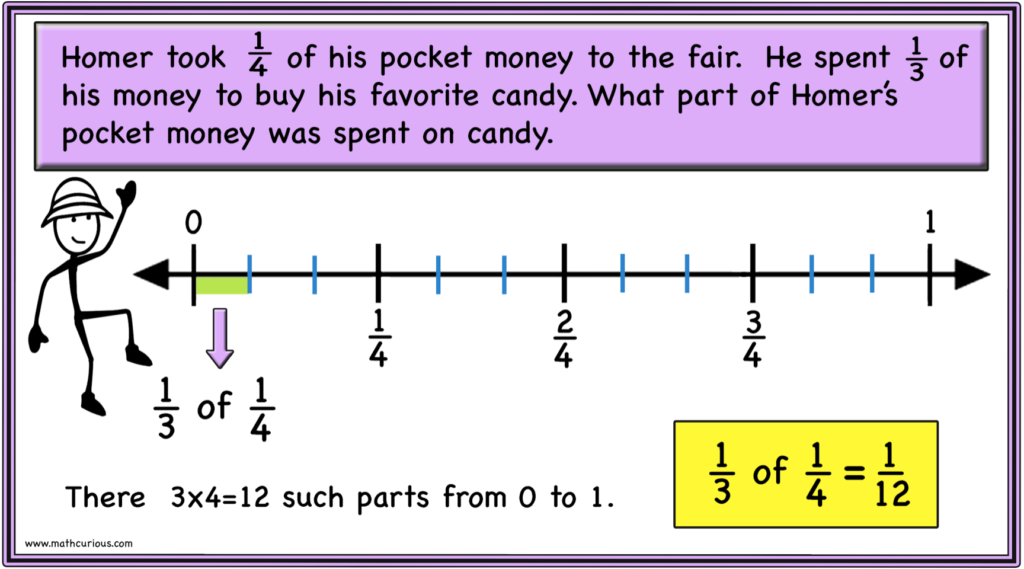
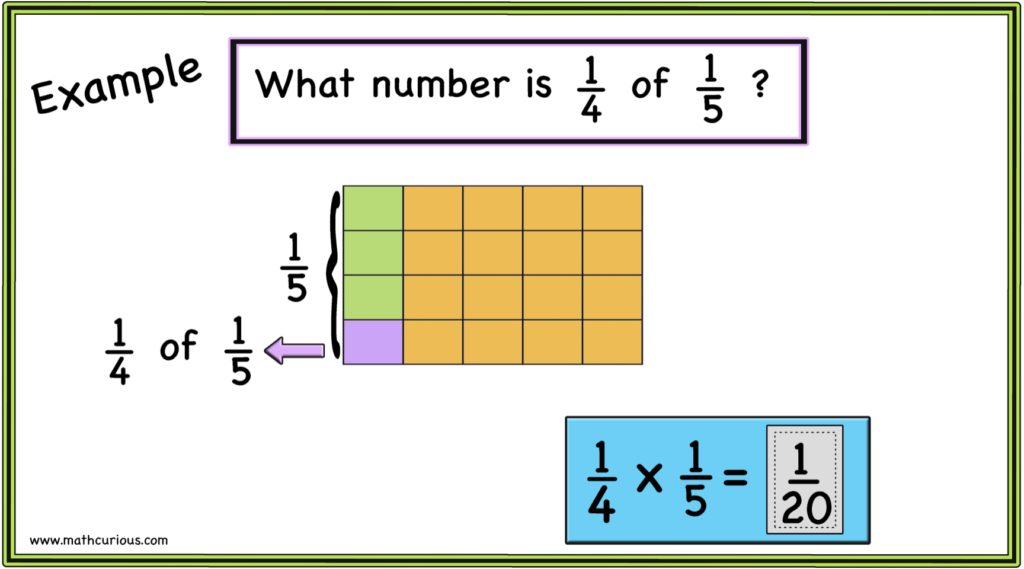
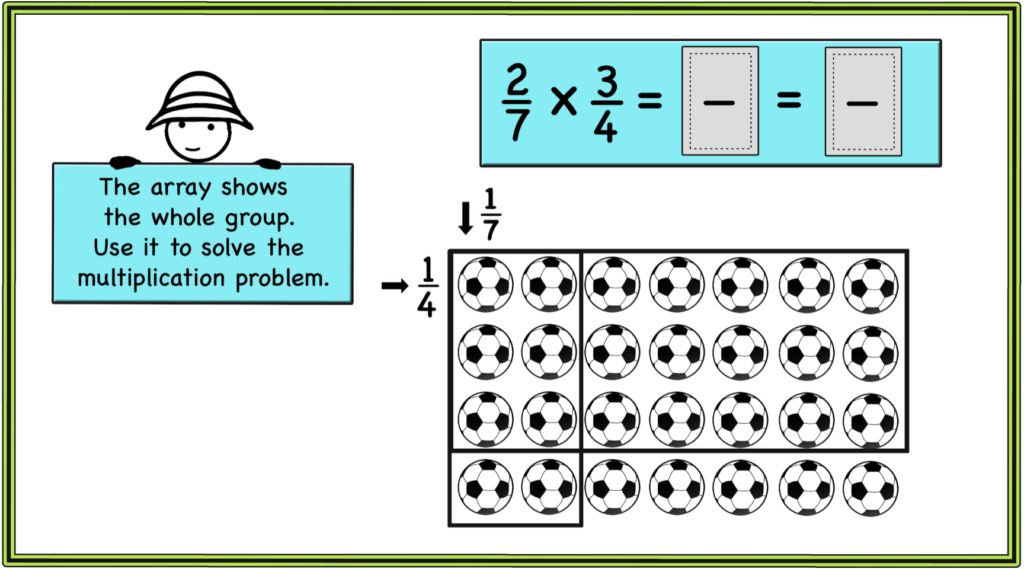
The students can color the parts or use transparent shapes.
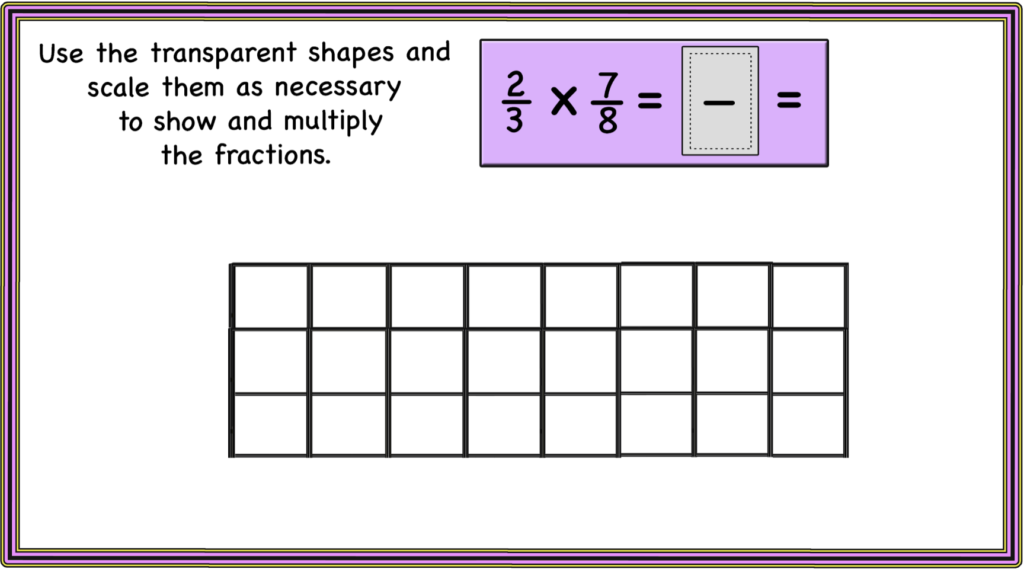
Once they understand the concept they will understand how the algorithm as well makes sense.
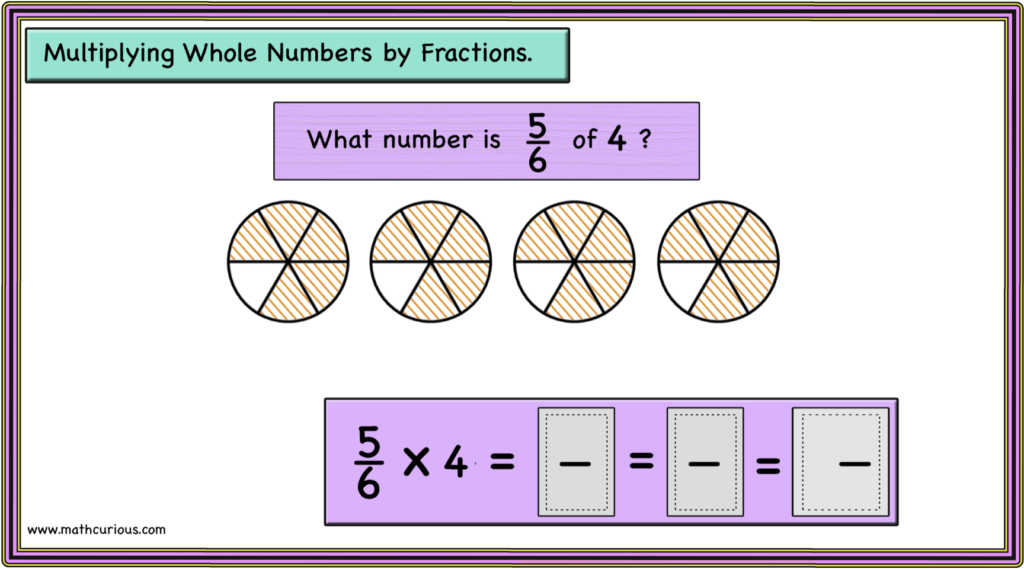
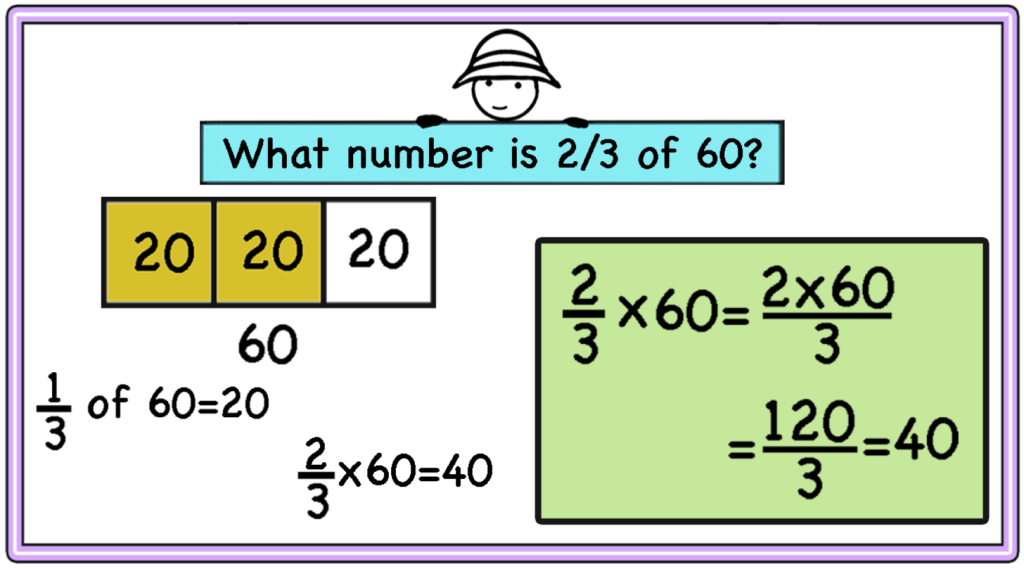
Multiplying by whole numbers
Multiplying fractions with whole numbers visually before using the algorithm is very important for understanding.
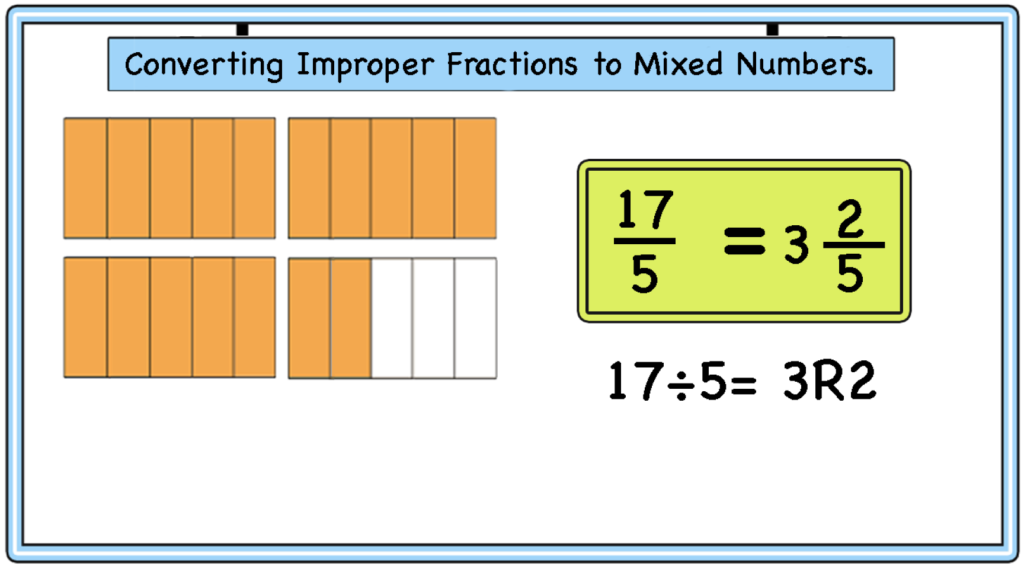
Converting Mixed Numbers to Improper Fractions
Multiplying mixed fractions requires the students to be able to convert mixed fractions to improper and the opposite. Provide visual examples and move on to faster computations.
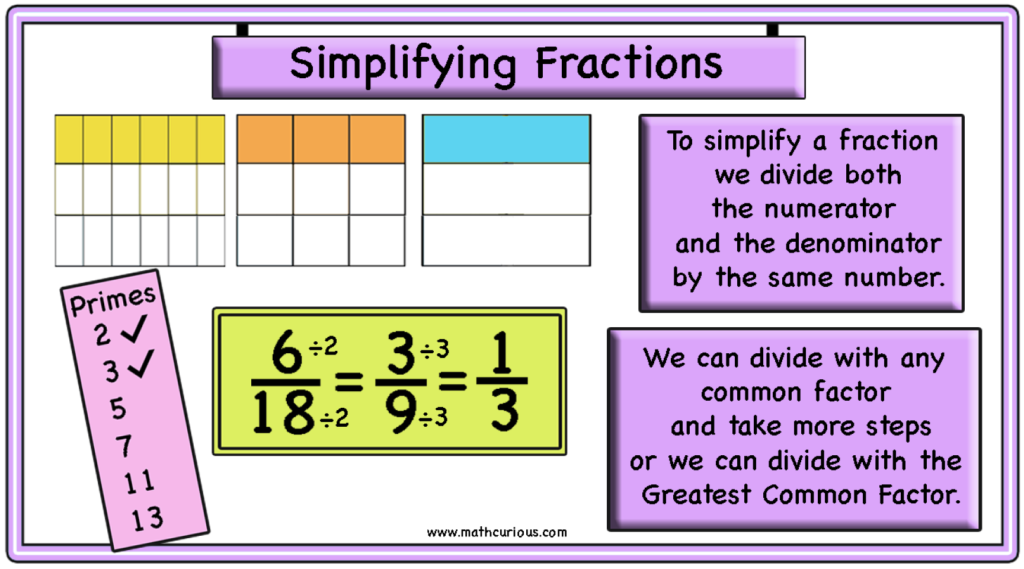
Simplifying Fractions
To simplify fractions students need to remember the concept of equivalent fractions.
They can use a list of prime numbers and check for each. This way they will take more steps to simplify the fraction to the simplest form.
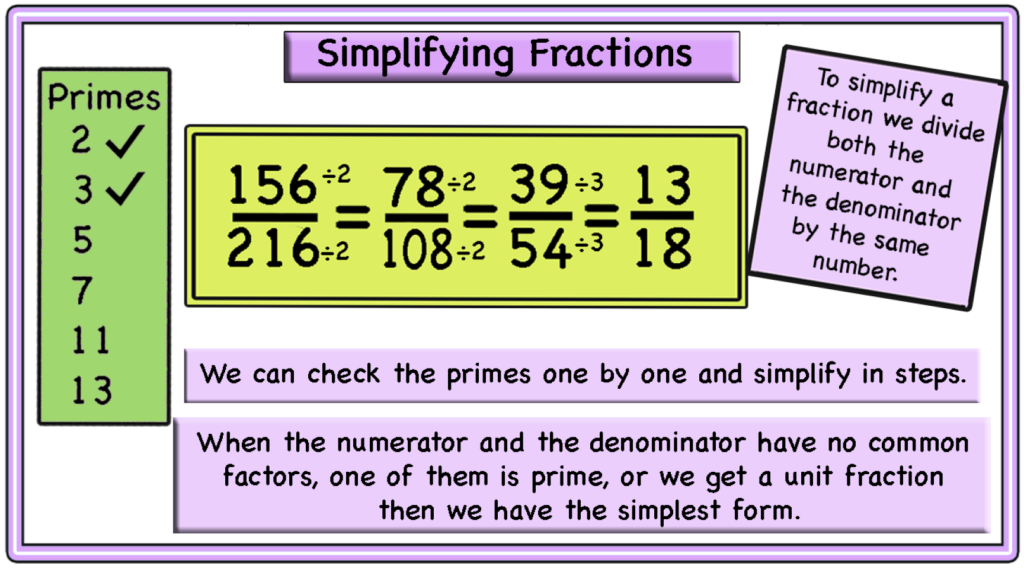
Another way is to find the Greatest Common Factor/Divisor and get to the simplest fraction in one step. They can use the multiplication table to find the GCF for smaller numbers or use prime factorization for bigger numbers.
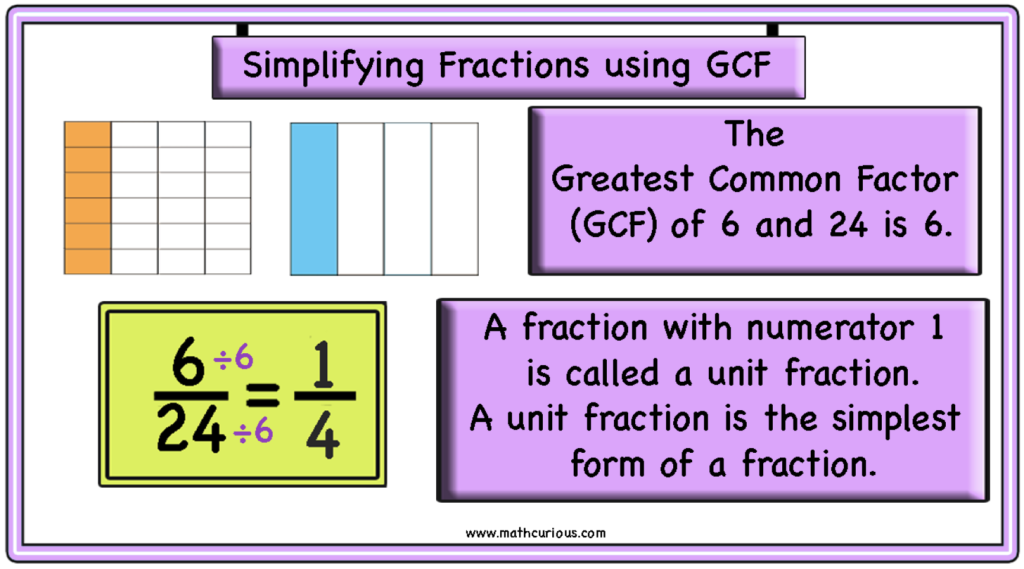
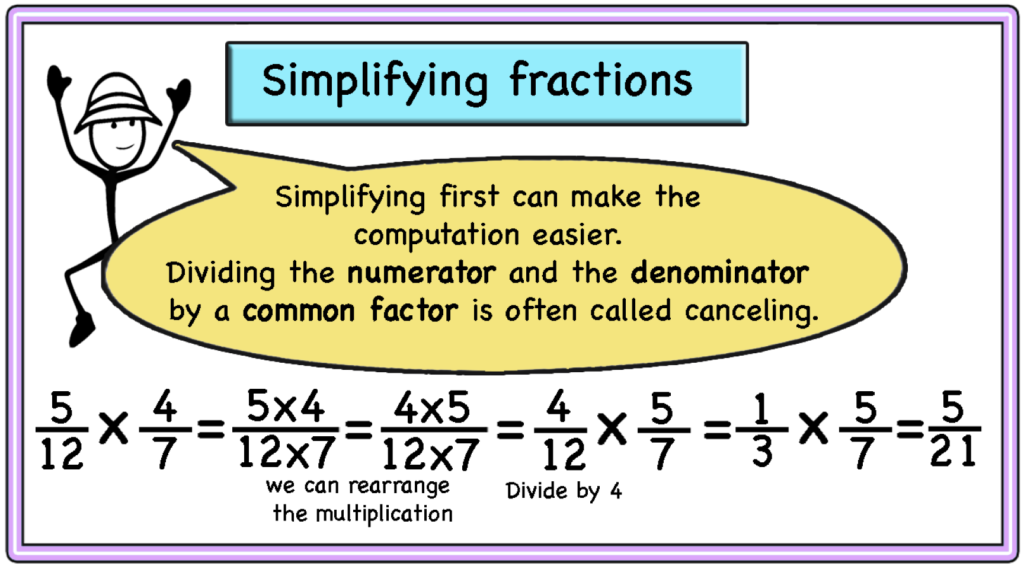
Simplifying fractions means finding an equivalent fraction with smaller numbers. It means that I need to divide the numerator and the denominator with the same (common) factor. Simplifying before multiplying is more efficient.
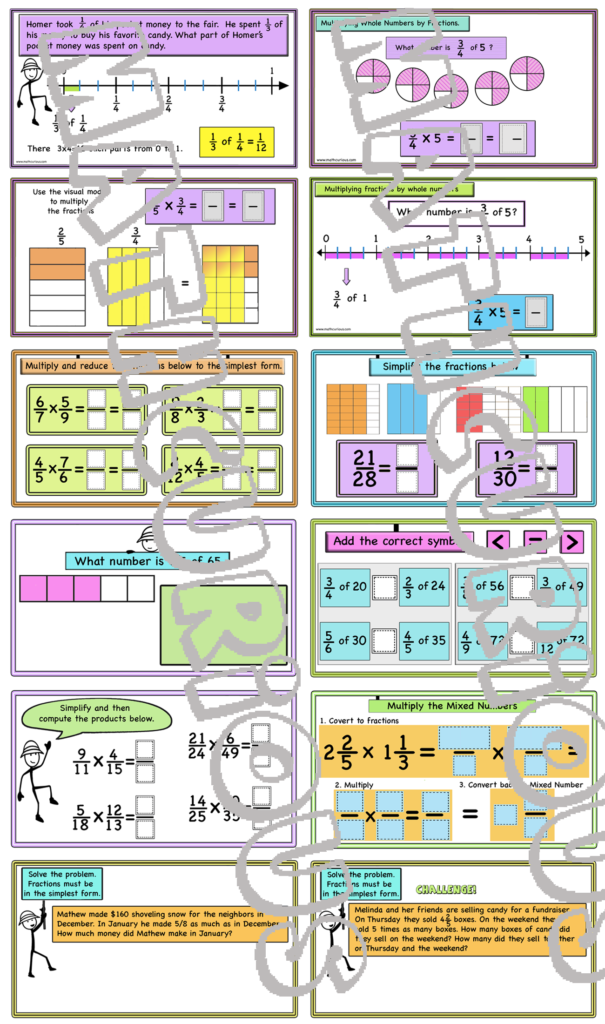
Word problems
Word problems are the best way to connect learning to the real world and show why we need to know how to multiply fractions. Allow the students to use a shape, diagram, and/or algorithm to show their understanding.

The resource includes 110 google slides activity cards and 110 print activity cards.
Find the bundle of the worksheets and the print and digital cards here
Find the bundle of Multiplication and Division of fractions print and digital activity cards here.